Advanced Option Pricing

(from Topics in Money and Securities Markets , Bankers Trust Company, Money Market Center)

INTRODUCTION

In an earlier paper, "Basic Option Pricing," Topics in Money and Securities Markets, July 1987, several methods for valuating options were presented. Chief among these was the binomial model. Its usefulness, as described in the earlier paper, was limited, in that the option was assumed to be a European call on a non-dividend paying stock. This paper will partially complete the analysis, providing extensions to the binomial to handle puts, American options, cash flows on the underlying asset, and options on assets other than stocks, as well as relax some of the assumptions made there.

AN EPIDEMIC OF EXTENSIONS

Valuing Puts with the Binomial

As a way of reviewing the binomial, let's present how to price European put options. with it. We are again assuming that

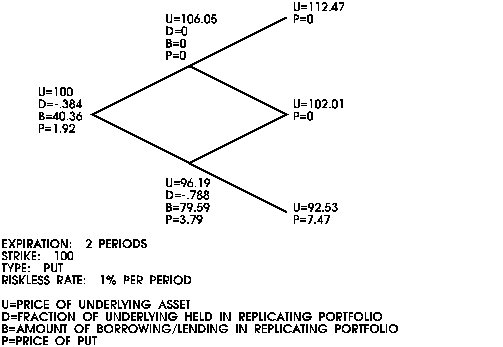
the underlying asset can go only up or down over a discrete period these jumps are known, and constant in size the risk free rate is known and constant we can borrow or lend at this rate there are no taxes or transaction costs we can buy and short the underlying asset, in any amounts the underlying asset pays no dividends over the life of the option

Given the price of the underlying today, as well as the jumps that may be achieved, we can draw a tree of all the possible values the asset can take on from today until expiration. Such a tree is shown in Figure 1, where the underlying is worth 100 today.

Figure 1: Possible Price Paths Over 2 Periods of an Asset Priced at 100 Today

Our goal is to price the option in the base node of the tree, today. While we cannot do that directly, we can simply determine the value of the option in the three nodes, or states of the world, that can exist at expiration. In this case, since we are valuing a put, the intrinsic value of the option at expiration is equal to

max(0,strike price - underlying price) {1}

We will fill in the values of the put with 100 strike in each of the three nodes, based upon {1}, and have Figure 2.

Figure 2: Possible Values of a 100 Put After Two Periods, with Associated Underlying Asset Prices

Now let's look in the node where the underlying is worth 96.19 (one period from now). The put can have two values after that, 0 and 7.47. What we are going to do is construct a portfolio in that node, with underlying at 96.19 and borrowing and lending allowed at 1% per period, that will be worth 0 if the underlying goes up, and 7.47 if the underlying goes down. This portfolio will have D shares of the underlying, and lend B dollars. To calculate D and B, we must solve:

102.01 + B (1+.01) = 0 {2a}

92.53 D + B (1+.01) = 7.47 {2b}

This says that the portfolio value after an up move (the left hand side of {2a}) must equal the put value after an up move (the right hand side of {2b}, and the portfolio value after a down move must equal the put value after a down move.

With two equations, and two unknowns (D and B) we can solve {2}quite easily. The value of this portfolio of D and B is always going to be worth the same as the option in that node, as well. This value

96.19 D + B {3}

is the value of the option when the underlying is worth 96.19.

We can apply this reasoning to get an option value in all the nodes in the second to last period (see Figure 3).

Figure 3: Underlying Asset, Replicating Portfolio and Option Values

But knowing those put values allows us to create a portfolio in each node in the preceding period that will replicate the option, and therefore have the same value in those nodes, as well. We can use this all the way back to the base of the tree, and say the value of the replicating portfolio using today's prices is the value of the put option.

Given all that, what was the difference between the way we priced puts and calls? Only the formula we use to get the intrinsic value of the relevant option in the last period of the tree. For the put, we used

max(0,strike price - underlying price) {1}

whereas a call would have used

max(0,underlying price - strike price) {4}

Combination positions

We can extend this reasoning to make the pricing model even more flexible. Suppose we want to value a portfolio of two call options with the same expiration, but different strikes. Rather than use our binomial model twice and add the answers together, we can save ourselves some time and effort by building one tree of underlying values, and then at expiration calculate the intrinsic value of the combined position. We then work out what portfolio would replicate the total cash flows. The portfolio value today that replicates the flows must be worth the same amount as the portfolio of two options.

Following this reasoning, we can also include puts (via {1}) as well as positions in borrowing or lending, or positions in the underlying asset, as part of the portfolio we are trying to replicate. If we include borrowing and lending of $x today, the tree will contain the future value of x in each node at expiration. If we are replicating the underlying asset, it is worth the value of the underlying asset in that node, of course.

For example, if we had a position that was long a 110 call, long a 95 put, long the underlying and long 50 par amount of a bill maturing at the options' expiration, then a table of intrinsic values of this position can be obtained (shown in Table 1).

Table 1: Valuing a Portfolio at Expiration

Underlying at Expiration Call Put Underlying Bill Total 85 0 10 85 50 145 90 0 5 90 50 145 95 0 0 95 50 145 100 0 0 100 50 150 105 0 0 105 50 155 110 0 0 110 50 160 115 5 0 115 50 170 120 10 0 120 50 180

By extending this table, and making the increments finer, we could value the whole portfolio in each node at expiration once and only once, and then work out what portfolio today would replicate that value at expiration.

Put-call Parity

One thing to notice about valuing puts and calls is that whichever equation is used, {1} or {4}, the intrinsic value of the option is never negative. We knew this already, that an option will never be exercised if it is out of the money. Since we have shown in an earlier paper that the binomial ends up giving us a weighted average of the intrinsic values, we can see that the option values it returns, weighted averages of all non-negative numbers, will conform to rational economic theory.

We can look at {1} and {4} another way, too, to derive an important relationship between put and call prices, named "put-call parity."

Imagine all the nodes the binomial tree generates at the expiration of the option. For a given strike price, in each node the underlying price is either above, at, or below the strike. This means that in each node, either {1} or {4}, but not both, is positive. In one special case, where the underlying in a node is equal to the strike, they'll both be zero. Now suppose you have a long position an a call, and a short position in a put, both with the same strike. The value of your total position at expiration in the nodes that are above the strike is

underlying asset - strike

and in the nodes below the strike is also

underlying asset - strike.

This is true no matter what the strike is, as long as it's the same for both the put and the call. Let's suppose you also buy a bill maturing at the expiration of the option, which will have a maturity value of the strike price. Combined with the put and call, the value of this position will be exactly equal to the underlying asset at expiration. Finally, if you short sell the underlying asset today, then at expiration your value will net out to zero. So a portfolio of

long call + short put + short underlying + lend present value of strike

will be worth zero in every state of the world at expiration. Therefore, it should not have any value today to avoid arbitrage. Thus, put-call parity states that

put = call - underlying + present value of strike{5}

If you want to use the call valuation model, applying {5} will generate a put value (as long as all the above assumptions are met, particularly the fact that we are valuing European options).

Options on Futures

In valuing options on futures, we need to adjust our algorithm slightly, but the changes are surprisingly minor. First, the stock price had to have up and down moves which surround the risk free rate to avoid dominance. However, a futures contract doesn't require money be put up that could be earning money elsewhere. Therefore, the up and down moves on a futures contract ought to surround a zero rate of return (we may have to put up margin, but since we can keep the interest the margin earns, we don't lose the use of the money). With a multiplicative process, as we have used before, this would lead, for example, a futures currently prices at 50 to rise to 50 x 1.02 or fall to 50/1.02.

The next change is in the two equations and two unknowns. We still want to make the value of the replicating portfolio be the same as the value of the option in each node, but owning a futures contract causes a marking-to-market profit/loss, and also the futures position itself has no value. Thus, holding D units of a contract, and lending B dollars, our new equations become:

D (F+ -F) + B (1 + R) = C+

D (F- -F) + B (1 + R) = C- {6}

where

F is the futures price in this node F+ is the futures price after an up move F- is the futures after a down move R is the one period interest rate C+ is the option price after an up move C- is the option price after a down move

Since the futures doesn't cost anything to hold, our final adjustment is to note the value of the replicating portfolio is just B.

Even with these changes, the value of D is still

{7}

which makes sense, as this is the same form as the formula for the derivative of C with respect to F, the basis for hedging (the derivative is taken over a change in the underlying, one binomial period hence. As the number of periods increases, this approaches the instantaneous derivative).

The formula for B, and thus the option value, is

{8}

Just as in the binomial model on an asset, the option value in a node is the present value of the up and down option values that can be reached from that node, weighted by pseudo-probabilities (numbers which sum to one, and are both between 0 and 1).

Figure 4 presents a simple tree that demonstrates the pricing of a call option on a futures contract.

Figure 4: An Option on Futures Example

Options on Forwards

In an efficient market, without stochastic interest rates, a forward and future on the same commodity with the same delivery date should be priced the same. (Although the futures will have intermediate cash flows due to marking-to-market, that can be hedged by holding, with T years to delivery and a rate r to delivery, 1/(1+r){to the T power} futures contracts for every forward to be hedged. This strategy will lead to the same position value at delivery as holding one forward contract.) Therefore, to value an option on a forward, we start by building the tree of underlying values exactly the same as we do for a futures option, as above. The only difference is that, in the next step, our two equations in two unknowns must take into account that, in current dollars, the profit/loss from the forward must be discounted back from delivery to the node in which we are solving the equations:

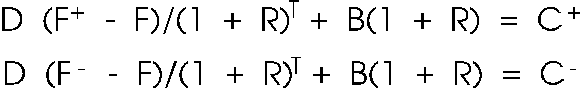{9}

where T is the time from the period after the underlying has moved to the delivery of the forward contract.

As with futures, the cost of the replicating portfolio is B, since the forward contracts held have no value today. This time, D will be:

{10}

which again is perfectly reasonable from a hedging standpoint, since the profit on the forward itself must be discounted to the present. The formula for B, interestingly enough, reduces to:

{11}

which is the same as the value of the option on a futures contract. This is sensible; since all the F's are the same, and the intrinsic values at expiration are the same, then the C+'s and C-'s are all the same.

In Figure 5,. a tree to value a call option on a forward is shown.

Figure 5: An Option on Forward Example

American Options

So far, all the options have been European. To turn any of them into American options models, we need only recognize one very simple rule: an American option can never trade for less than intrinsic value. If, during our walk backwards through the tree, we come up with a replicating portfolio that is worth less than intrinsic value, we substitute intrinsic value for the option in that node, and know that the option will be exercised at that point.

This may seem somewhat simplistic and arbitrary. After all, the replicating portfolio is supposed to yield portfolio values to match the option, so why should we adjust afterwards? The reason is that the construction of the replicating portfolio assumes the option will exist in the next period. But any time the intrinsic value of the option is more than this amount, the option holder is looking at holding an instrument whose value next period can be replicated more cheaply by holding other assets. Holding the option seems useless. He could try selling the option, but no one will pay more than intrinsic value (since next period they will be left with something worth less than the intrinsic value today), so the only way out of the dilemma is to exercise the option immediately. At this point, if he were to sell, instead, he would be indifferent between exercising or selling at intrinsic value.

To recap, as we work through the tree, after solving for the cost of the replicating portfolio, we make the option value OV

OV = max(replicating portfolio value, intrinsic value) {12}

Looking at this from another angle, we can also see the obvious rule for exercising an option early; only exercise when the option is trading at the intrinsic value. If it has any time premium (the excess over intrinsic value) you could realize more by selling the option.

A classic example of this is the proof that it is never advantageous to exercise an American call on a non-dividend paying stock early. Suppose you are long a call option with strike price K. The underlying is worth S now, and is in the money. If you exercise now by borrowing the strike at rate R to expiration you will have the underlying less K x (1 + R) with certainty. On the other hand, if you wait until expiration, you will have the underlying less K if the call is in the money, and 0 otherwise. Either way, you are ahead by waiting until the last minute to exercise your call.

One thing that we lose with American options is the ability to use the simple expected value formula to price the option. Now, not all the nodes of the tree will be reached, if there's a possibility of early exercise. The expectation must then use a complicated conditional distribution.

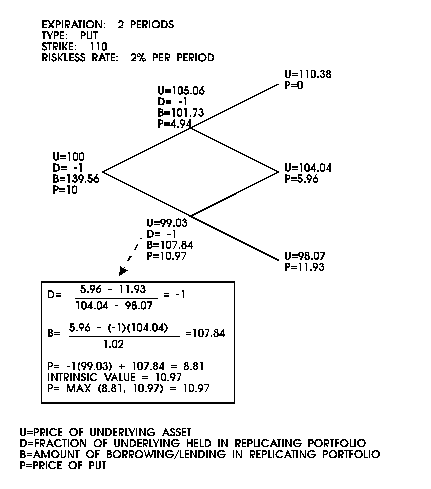
Figure 6 gives an example of pricing an American put option.

Figure 6: An American Option Example

Cashflows on the Underlying Asset

Another major restriction we've imposed so far is that there be no cashflows on the underlying asset. This leaves out dividend paying stocks and even spot foreign exchange. (It does not leave out most options on debt instruments, even those with coupons. These are "cash flow protected" in that the strike price at a given time reflects the accrued interest on the exercise date, which means the amount that must be paid to exercise a call before a coupon date is more (by the amount of the coupon) than what it costs to exercise the call right after a coupon is paid.) We will now remedy that.

In the absence of taxes, if the only uncertainty concerns the price of the underlying asset next period, an instrument's total return over a day ought to be the same whether a cashflow was paid or not:

{13}

This means that if the cashflow is 2% one day, then the price should drop by 2% to reflect that (net of other factors). For a stock, the firm has lost value by an amount equal to the cash distribution.

To keep the same return process as we've already used for the binomial, what we will do instead is, in those periods when a cash flow is to be made, make the up price be:

S+ = S x u - cash flow+ {14}

and the down price

S- = S x d - cash flow-

where

S is the price in the node before the jump

u is the size of the up jump for an equivalent, non-cash paying asset

d is the size of the down jump for an equivalent, non-cash paying asset

This assumes we know what the cash flow will be, and when. For stocks, this will most likely mean choosing periods closest to quarter year intervals (when dividends are paid), to use the special form of {14}. For foreign exchange, where the option is on spot currency, we might assume that it is earning a daily coupon equal to the short term rate in that currency.

In making a statement about the size of the cash payment, we can calculate it in a variety of ways. We can have a forecast of dividends in dollars, for instance the dividend stream can be 2, 2.2, and 2.42 over the option's life. We can also be a little fancier, and make the dividend be a percentage of the asset's price in each node. This would lead to higher dividends for higher stock prices. In fact, we can have any type of function of the stock price we want, so long as it is a function of just the stock price and the time period, and not other factors we have not included in our model. Note that if we are going to pick certain arbitrary dividend patterns, we may have to use the bushy tree version of the binomial, described in the section on non-constant volatility.

Armed with a new way of building the tree, we will continue to calculate the option value by looking at the intrinsic value at expiration (using these new, cash flow affected prices of the underlying in the tree). Our two equations in two unknowns will recognize that holding a portion of the underlying may give us a cash flow, as well:

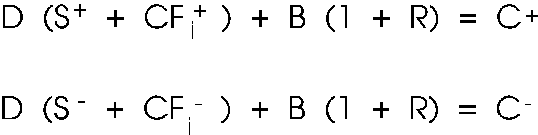{15}

where CFi + (CFi-) is the cash flow in the ith binomial period, after an upmove (downmove). We have CFi here, unlike before, but since it is known we can still solve for D and B as easily as before. Once again, the option value is the value of the replicating portfolio, D x S+B.

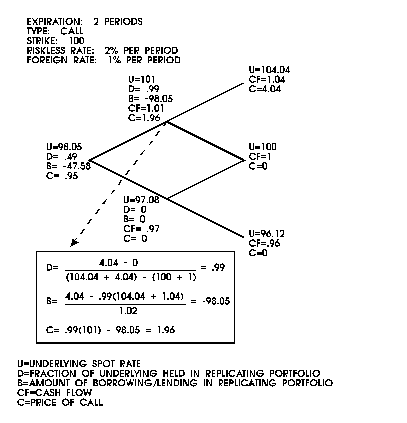
Figure 7 shows an example of valuing a call option on spot FX, which assumes a cash flow each period.

Figure 7: An FX Option Example

European/American Options

Some options are hybrids of American and European. They are exercisable on certain dates, but not on others. The prime example of this is the call option embedded in many bonds. They are not callable for the first few years after issue, but after that they may be exercised any time up to maturity. These are very simple to handle with the binomial. Merely invoke {12} only in those periods after the first call date: if the option is American in that period, make sure it is worth at least intrinsic value. If it is European in that period, the option is worth the value of the replicating portfolio.

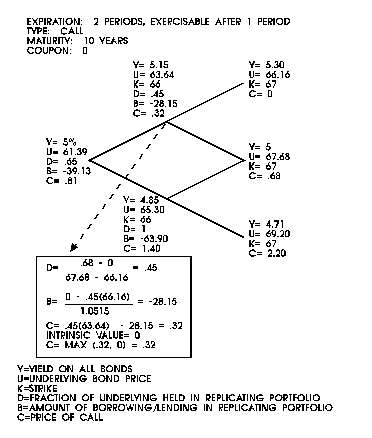
In Figure 8, a bond call option example is shown, with a changing strike, that is not exercisable in the first period.

Figure 8: A Hybrid American Bond Option Example with Changing Strike

Options on Bonds

In an earlier paper, we introduced a way to value options on bonds using the binomial model (see "Assessing the Value of a Callable Eurobond," Topics in Money and Securities Markets, July 1987). We will quickly restate the idea here. First, we want to point out why it is important to cast the model the way we do.

When we apply the binomial to a stock, we first place the current price in the base node. We then apply the up and down moves to this price. As we go further out, we continually apply the same up and down moves. By the end of the tree, the price is widely dispersed around the current forward price of the stock. There are two reasons why this will not work well for bonds. First, as the bond approaches maturity, its duration declines, and with it its price volatility. Second, the bond's price must move towards par as it approaches maturity, whereas the stock price is unconstrained.

The simplest way around both these problems is to make the bond's yield be the stochastic variable in the model (the thing that moves up and down in the tree). If the yield on the bond today is 8%, we can posit it to go up to 8 x 1.05, or down to 8/1.05. We will then build the tree using this same 1.05 factor all the way through. When we are done, we'll have a tree of yields, but using the price-yield relationship we uniquely convert this to a tree of prices. The prices will have the property that they will all be converging to par, and the jumps in prices will be getting smaller as we move through the tree.

This is fine as an approximation, but it will turn out that this model has a number of flaws in it which are quite subtle and difficult to handle. Nevertheless, the prices that come out will still be fairly close to those gotten via a more sophisticated and correct model. It is beyond the scope of this paper to present an algorithm which addresses these other problems.

Figure 8 is an example of how to value a call option on a bond when the yield curve is assumed to be flat, and only moves in parallel. In it, the option is American after the first period, and the strike is assumed to change (see the next section).

Options With a Changing Strike

Certain options have their strike prices changing over their life. For an example of this, we can again turn to bonds. If the strike is quoted as the price such that the bond will yield 8%, then any bond with a coupon other than 8% is going to have a different strike price depending on when the option is exercised (this is sensible only if we are talking about American options). To handle this, we merely calculate the intrinsic value based upon the relevant strike before invoking {12}.

This is just an extension of the way we handled combination positions with one tree earlier Then, we valued two calls with different strikes at once. In general, we could have a complicated payoff diagram, and use the payoff at a given level of the underlying to determine the intrinsic value. In other words we have a function called intrinsic value, which is a function of the underlying price at expiration. IV(S) is a function of S, the underlying asset price. For a position that is long a 100 call and a 105 call,

IV(S) = max(O,S - 100) + max(O,S - 105) {16}

Now we are extending this idea to periods before expiration. We have IV(S,t) instead of merely IV(S). For a bond with a strike yield of 9%, if S is the yield in a given node of the tree, we might have:

IV(S,t) = max (O, price of bond with yield S and t years to maturity-price of a bond with yield 9% and t years to maturity). {17}

where t is the maturity of the bond in the binomial period for which the intrinsic value is being calculated. We don't want to extend the analogy too far, though. While it is possible to value many European options with the same expiration with a single pass through the tree by combining the positions, this is not something we can really take advantage of for American options. Now, while we can walk through the tree once, we must keep track of each option's value individually, which means we won't save very much time.

Figure 8 shows an example of a call option on a bond with a yield strike.

Including Taxes

So far we have assumed there is no such thing as taxes. Unfortunately, that's not the case. Including them in a pricing model opens a Pandora's box, which is the second most important reason we don't like them.

Taxes make our replication path dependent. This term means that the value of the hedge portfolio at expiration is a function of how the underlying got there, and not just where the asset ends up. The path dependence is a result of paying taxes on realized gains, and not paper profits. For instance, if the underlying goes up, the delta on a call will rise, and we will buy more of the underlying asset. Afterwards, when it falls, we sell the underlying and pay taxes based upon the price or prices at which we initially bought. Instead, if the underlying falls, we will sell after only one period and pay taxes at that time (or, to complicate matters more, we may not have to pay taxes for another year, which means it's the present value of the tax that we pay). After that, when the underlying rises, we will be buying, and not pay taxes at that point. So while the underlying asset has the same value whether we go up down or down up, the tax payments are different in each path.

Taxes can also be different on different types of transactions. While we no longer have preferential tax treatment for capital gains in the U.S., that is not true worldwide. And it may not hold forever, here, either. We need to know the tax status of the individual or institution trading the option. We need to know whether they have other income to offset losses incurred in the option or the hedge portfolio. If they apply existing positions to either side of the hedge transaction it may be an old trade eligible for long term capital gains. Futures may be taxed differently form stocks, which may be different from interest, which may depend on whether the borrowing or lending was done on an original issue discount basis. Taxes vary from state to state, and for an option the tax liability may depend on what type of business the user is in. In short, it is going to be difficult, if not impossible, to incorporate all this into a pricing model.

So let's simplify. Assume all gains are taxed at the same rate, and all losses are deductible against other gains. Assume also that all taxes are payable immediately (including tax on other income, so losses are deductible immediately). We will see that taxes drop out of the binomial model completely. The intuition for this is that the option holder is taxed the same as the replicator, so the amount of money the replicator needs at expiration is the same on a pre-tax basis as before. We will now present a more rigorous proof of this.

First, we will build our price tree as before. Our two equations in two unknowns, though, will reflect gains and losses. What we need is for the after-tax value of the replicating portfolio to be worth the same as the after-tax value of the option at the end of the period in both the up and down states. Let's look at the next-to-last period. We know in the last period what the pre-tax value of the option will be in each node, applying the tax rate gives C+ and C-. We also know the value of the underlying in that node, S, as well as the underlying price in the up and down node,

{18}

where t is .5 at a 50% tax rate.

Notice that this down state leaves us better off than before we had taxes, because we assume that the tax loss lowers other tax liabilities we have. In general, our two equations in two unknowns become:

{19}

Since C is D * S = B, this becomes:

{20}

Subtracting one from the other,

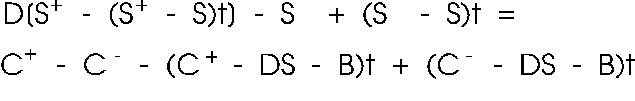{21}

so

{22}

{23}

Equations {22} and {23} match the old formulas for D and B exactly. Not only that, but the intrinsic value at expiration is the same no matter what the tax rate is. While this may seem unintuitive, no one would ever sell an option for less than intrinsic value, whether they're getting a piece of their loss back from the government or not. This means that the value of an option is the same, independent of the tax rate (given our simplifying assumptions).

Allowing Non-constant Interest Rates

One of our assumptions was that the riskless rate was known and constant over the life of the option. This meant that the yield curve was flat, and was not going to move at all. We will keep the yield curve from moving, but will now allow it to have some slope or curvature.

Given a yield curve, we can back out the forward rate from any point in the future to any other point. In building the binomial tree, the first period is from today (t0) to some point t1 in the future. The next period goes from t1 to t2, and so on until we go from tn-1 to tn, where n is the number of periods in the tree and tn is the expiration of the option. For the first period in the tree, we should use the forward rate from t0 to t1 as the riskless rate at which we can borrow and lend. For the next binomial period, we'll use the forward rate from t1 to t2, and follow this scheme all the way to expiration.

We will have an r{sub i} that represents the risk free rate over the ith binomial period. Now only will we use this rate in our two equations in two unknowns to borrow and lend, but it will also be important if we build our up and down moves around the mean return on the underlying asset, and that mean is a function of the risk free rate (from the Appendix to "Basic Option Pricing," we saw that one way to build a tree was to set

5 u = e µ/m + v/m

{24}

and

5 d = e µ/m - v/m

where µ is the annual log mean return on the asset

v is the annual log standard deviation on the asset

m is the number of binomial periods per year

This discrete stochastic process will converge to lognormal diffusion process (as n approaches infinity) if we set

µ = fr - v²/2 {25}

where rf is the one period risk free rate,

v is the one period log standard deviation of the underlying asset's return.

The proof of this follows from the Taylor series for e(x) of order 2. It may be seen intuitively since the binomial tree is multiplicative and open-ended on the upside, but bounded by zero on the downside. This means the more periods we have in the tree, the more upward growth there is. In the limit, with continuous returns, it is necessary to make the mean of the continuous process lower to compensate for this, and to make the mean over a finite interval equal the risk free rate. In actual fact, though, as we add more periods, this adjustment matters less and less. For the examples in this paper we ignore it for simplicity.)

Figure 9 shows a case where the yield curve is upward sloping.

Figure 9: Upward Sloping Yield Curve Example

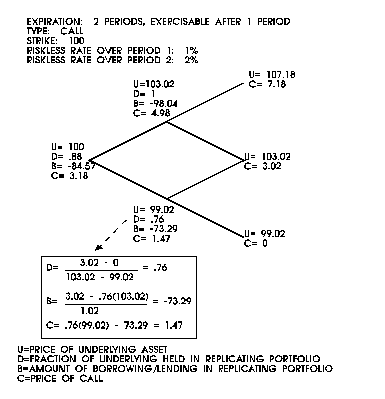
Non-constant Volatility

In every case so far, we have had trees that grew in size in a linear fashion: one node became two, two became three, and so on. This is a result of an up move followed by a down giving the same price as down and then up. One feature usually necessary for such a non-bushy tree is that the volatility be constant throughout the life of the option. For example, if an up move over the first period is 1.05, a down move 1/1.05, and in the second period the moves are 1.08 and 1/1.08, our paths will lead to:

{26}

from up down, and

{27}

from a down up path. Because these two don't match, we need to build a tree like the one in Figure 10.

Figure 10: A Bushy Tree

This problem can become computationally unfeasible very quickly. If a non-bushy tree with 25 periods takes 1 second to value, a bushy tree with 25 would take over a day, and 50 periods would take 100,000 years! Clearly, we want to avoid non-bushy trees whenever possible.

When we do have to use a bushy tree, though, we don't do anything differently, we just do a lot more of it. We build the tree assuming the asset can go up or down. Then we work out the intrinsic value at expiration. Then we work backwards through the tree, solving our two equations in two unknowns for the value of the replicating portfolio.

There are times when volatility can appear to be non-constant, and we can have a non-bushy tree. A great example of this is bonds again. The price volatility of a bond will decline as it approached maturity. If we build a tree in yields, as we did before, then convert each node's yield to a price, the price jumps will be declining in magnitude as time passes. The price movements will not in general be a simple function, even if the yield moves are.

SUMMARY

This paper has extended the basic binomial model presented earlier. It has discussed how to value puts, American options, American/European hybrids, options on futures, and forwards. It has also shown how to value options when there are cash flows on the underlying asset, a changing strike price, a sloping yield curve, and changing volatility. Taxes and options on bonds were discussed, as well as put-call parity. All in all, a lot of ground has been covered. But the binomial model has proven to be remarkably adept at handling each of these wrinkles. Once the basic idea is understood, that a portfolio that has the same cash flows as an asset will have in the future must have the same price as that asset today, it is very easy to change the equations slightly for each of the above features. Its simplicity is perhaps its greatest virtue. For while there may be other methods to value some of the options listed above, a strong grasp of the binomial will allow one to use it to value options that don't exist yet, as well.